20. Convergence of Positive Series
b.1. The Integral Test
a. Proof
If \(a_n=f(n)\) where \(f(x)\) is a continuous, positive, decreasing function on \([n_o,\infty)\), then \(\displaystyle \sum_{n=n_o}^\infty a_n\) is convergent if and only if \(\displaystyle \int_{n_o}^\infty f(x)\,dx\) is convergent.
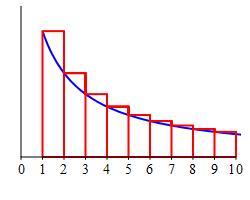
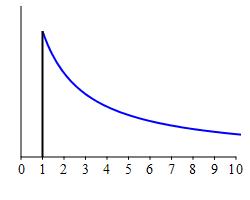
To be concrete, let \(n_o=1\), and assume \(f(x)\) is a continuous, positive, decreasing function on \([1,\infty)\). The graph of \(f(x)\) is shown at the right. The area under the curve is given by the integral \(\displaystyle \int_1^\infty f(x)\,dx\). Now let \(a_n=f(n)\). The series \(\displaystyle \sum_{n=1}^\infty a_n\) can also be regarded as an area, but in two ways. The term \(a_n\) may be regarded as the area of a rectangle whose height is \(a_n\) and whose width is \(1\). This rectangle may be positioned to the left or right of \(x=n\) resulting in the next two graphs. The series \(\displaystyle \sum_{n=1}^\infty a_n\) measures the total area of the red rectangles in either plot.
This figure shows \[ \sum_{n=2}^\infty a_n < \int_1^\infty f(x)\,dx \] Notice that we have dropped the first term of the series (with \(n=1\)) since the first red rectangle is not under the blue curve. Consequently, if \(\displaystyle \int_1^\infty f(x)\,dx\) is convergent (i.e. finite), then \(\displaystyle \sum_{n=2}^\infty a_n\) is also convergent. However, this is a tail of the original series. So, the series \(\displaystyle \sum_{n=1}^\infty a_n\) also converges.
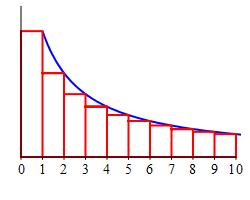
This figure shows \[ \int_1^\infty f(x)\,dx \lt \sum_{n=1}^\infty a_n \] Consequently, if \(\displaystyle \int_1^\infty f(x)\,dx\) is divergent (i.e. infinite), then \(\displaystyle \sum_{n=1}^\infty a_n\) is also divergent.